Complex Proportions

In this video, we will be learning how to solve complex proportions (variables on both sides) using cross-multiplication. After you finish this lesson, view all of our Algebra 1 lessons and practice problems.

Example of Solving a Complex Proportion

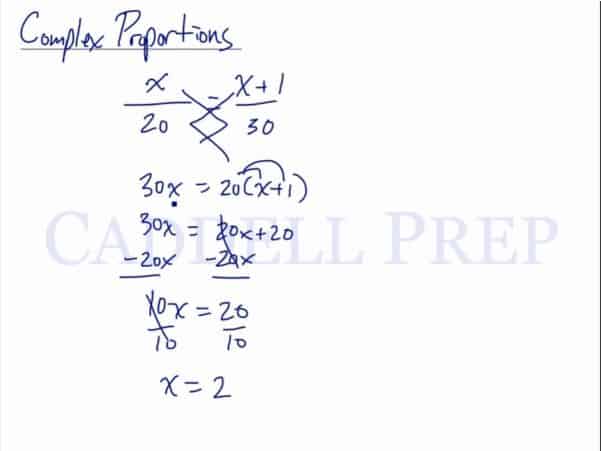
\dfrac{x}{20}=\dfrac{(x+1)}{30}\leftarrow First we cross-multiply

30x=20(x+1)\leftarrow Then we distribute the 20 to the x and 1

30x-20x=20x +20-20x\leftarrow Subtract 20x from both sides to isolate x

\dfrac{10x}{10}=\dfrac{20}{10}\leftarrow Divide by 10 on both sides

x=2

Complex Proportions

Example 1

\dfrac{3x}{10}=\dfrac{2(x+5)}{15}

First, distribute 2 to each of the terms inside the parenthesis

\dfrac{3x}{10}=\dfrac{2x+10}{15}

Then, cross multiply

15(3x)=10(2x+10)

Distribute 10 to each of the terms inside the parenthesis

45x=20x+100

Subtract 20x from both sides to isolate x

45x-20x=20x+100-20x 25x=100

Divide by 25 on both sides

\dfrac{25x}{25}=\dfrac{100}{25}

Therefore,

x=4

Example 2

\dfrac{3x+6}{5}=\dfrac{3(x+4)}{6}

First, distribute 3 to each of the terms inside the parenthesis

\dfrac{3x+6}{5}=\dfrac{3x+12}{6}

Then, cross multiply

(6)(3x+6)=5(3x+12)

Distribute constants to each of the terms inside the parenthesis

18x+36=15x+60

Subtract 15x from both sides

18x+36-15x=15x+60-15x 3x+36=60

Subtract 36 from both sides to iolate [latex]x

3x+36-36=50-26 3x=24

Divide 3 on both sides

\dfrac{3x}{3}=\dfrac{24}{3}

Therefore,

x=8

Video-Lesson Transcript

Let’s go over complex proportions.

For example:

\dfrac{x}{20} = \dfrac{x + 1}{30}

What makes this complex is that we have one variable on both sides of the equation.

We’re just going to do the same method which is to cross-multiply.

x \times30 = 30x

To solve the equation on the right, we have to distribute 20 on each of term.

{x + 1} \times20 20x + 20

Now we have

30x = 20x + 20

Let’s get all the variables on one side by subtracting 20x on both sides.

30x - 20x = 20x - 20x + 20 10x = 20

Simply solve x by dividing both sides by 10.

\dfrac{10x}{10} = \dfrac{20}{10}

And we’ll have

x = 2

Complex proportion is different from regular proportion because it has a variable on both sides of the equation.