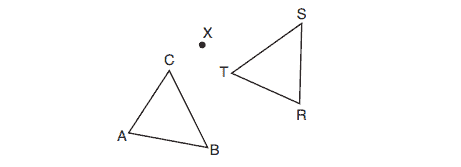
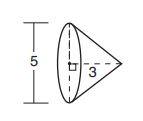1. After a counterclockwise rotation about point , scalene triangle
maps onto
, as shown in the diagram below.
Which statement must be true?
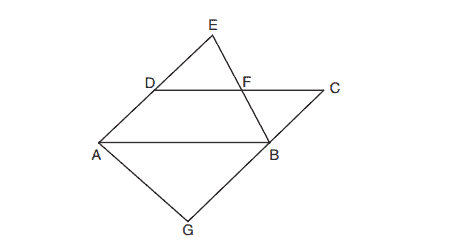
(1)
(2)
(3)
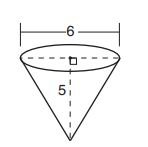
(4)
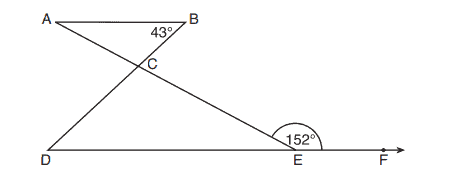
2. In the diagram below, ,
, and
at
,
, and
.
Which statement is true?
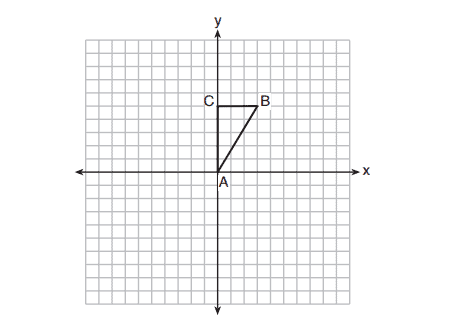
(1)
(2)
(3)
(4)
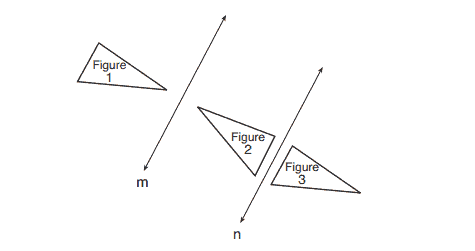
3. In the diagram below, line is parallel to line
. Figure 2 is the image of Figure 1 after a reflection over line
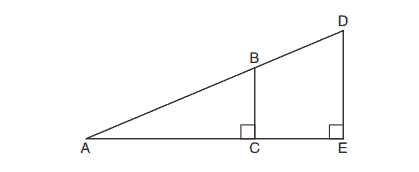
. Figure 3 is the image of Figure 2 after a reflection over line
.
Which single transformation would carry Figure 1 onto Figure 3?
(1) a dilation
(2) a rotation
(3) a reflection
(4) a translation
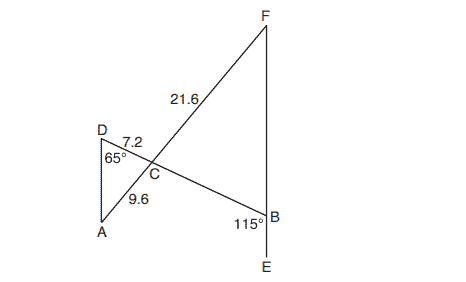
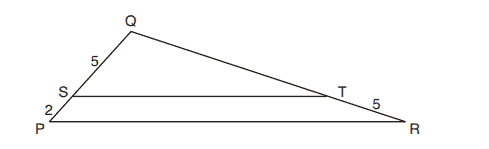
4. In the diagram below, and
intersect at
, and
and
are drawn such that
,
,
,
, and
.
What is the length of ?
(1)
(2)
(3)
(4)
5. Given square , where
cm. If square
is dilated by a scale factor of
about a given center, what is the perimeter, in centimeters, of the image of
after the dilation?
(1)
(2)
(3)
(4)
6. In right triangle , hypotenuse
has a length of
cm, and side
has a length of
cm. What is the measure of angle
, to the nearest degree?
(1)
(2)
(3)
(4)
7. The greenhouse pictured below can be modeled as a rectangular prism with a half-cylinder on top. The rectangular prism is feet wide,
feet high, and
feet long. The half-cylinder has a diameter of
feet.
To the nearest cubic foot, what is the volume of the greenhouse?
(1)
(2)
(3)
(4)
8. In a right triangle, the acute angles have the relationship . What is the value of
?
(1)
(2)
(3)
(4)
9. In the diagram below, ,
, and
and
are drawn.
Which statement is always true?
(1)
(2)
(3)
(4)
10. The base of a pyramid is a rectangle with a width of cm and a length of
cm. What is the height, in centimeters, of the pyramid if its volume is
cm
?
(1)
(2)
(3)
(4)
11. In the diagram below of right triangle ,
.
Which statement is always true?
(1)
(2)
(3)
(4)
12. What is an equation of the line that passes through the point () and is perpendicular to a line with equation
?
(1)
(2)
(3)
(4)
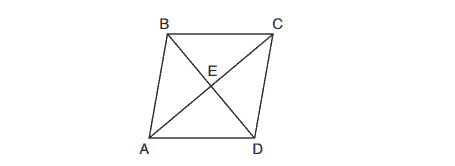
13. The diagram below shows parallelogram with diagonals
and
intersecting at
.
What additional information is sufficient to prove that parallelogram is also a rhombus?
(1) bisects
(2) is parallel to
(3) is congruent to
(4) is perpendicular to
14. Directed line segment has endpoints
and
. Point
divides
such that
is
. What are the coordinates of
?
(1) ()
(2) ()
(3) ()
(4) ()
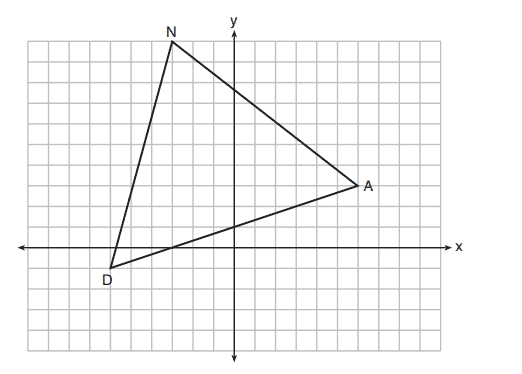
15. Triangle is graphed on the set of axes below. The vertices of
have coordinates
,
, and
.
What is the area of ?
(1)
(2)
(3)
(4)
16. Triangle , with vertices at
,
, and
, is graphed on the set of axes shown below.
Which figure is formed when is rotated continuously about
?
(1)
(2)
(3)
(4)
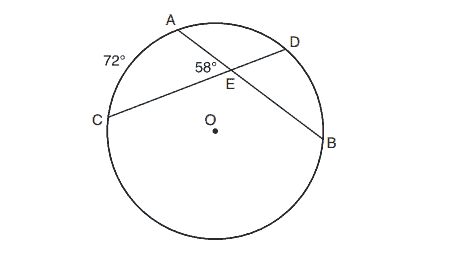
17. In the diagram below of circle , chords
and
intersect at
.
If and
, how many degrees are in
?
(1)
(2)
(3)
(4)
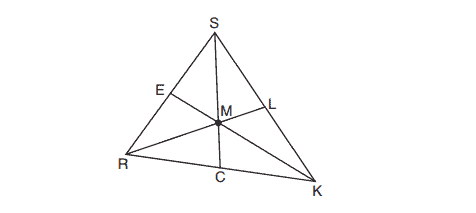
18. In triangle below, medians
,
, and
intersect at
.
Which statement must always be true?
(1)
(2)
(3)
(4)
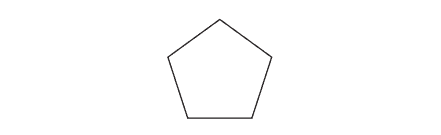
19. The regular polygon below is rotated about its center.
Which angle of rotation will carry the figure onto itself?
(1)
(2)
(3)
(4)
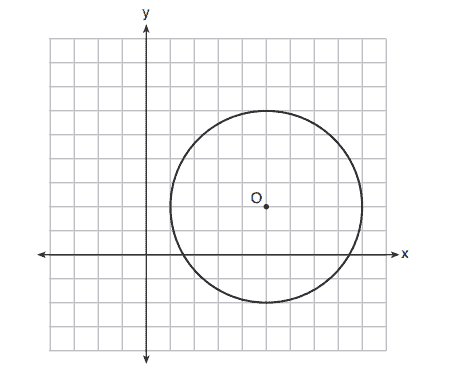
20. What is an equation of circle shown in the graph below?
(1)
(2)
(3)
(4)
21. In the diagram below of ,
is drawn parallel to
,
,
, and
.
What is the length of ?
(1)
(2)
(3)
(4)
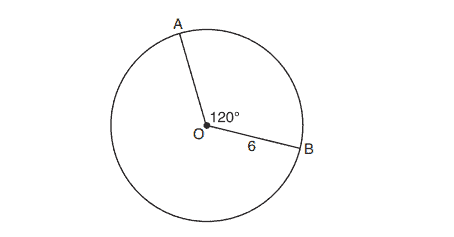
22. The diagram below shows circle with radii
and
. The measure of angle
is
, and the length of a radius is
inches.
Which expression represents the length of arc , in inches?
(1)
(2)
(3)
(4)
23. Line segment is the altitude drawn to hypotenuse
in right triangle
. If
and
, then, to the nearest tenth,
is
(1)
(2)
(3)
(4)
24. Line is dilated by a scale factor of
centered at the point (
). If
is represented by
, which equation can represent
, the image of
?
(1)
(2)
(3)
(4)