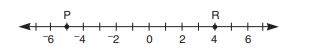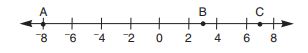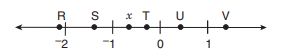51.
A.
B.
C.
D.
E.
52. If of
is
, what is
of
?
F.
G.
H.
J.
K.
53. If and
, what is the value of
?
A.
B.
C.
D.
E.
54. Carlos is picking colored pencils from a large bin that contains only red pencils,
green pencils, and
blue pencils. Without looking, Carlos pulls out
pencils. If the pencils were distributed randomly in the bin, how many pencils of each color is it most likely that he picked?
F. red,
green,
blue
G. red,
green,
blue
H. red,
green,
blue
J. red,
green,
blue
K. red,
green,
blue
55. How many positive integers satisfy the inequality ?
A.
B.
C.
D.
E.
56.
F.
G.
H.
J.
K.
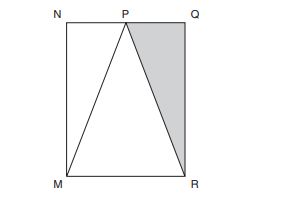
57.
In the figure above, the base of is a side of rectangle
, and point
is the midpoint of
. If the area of the shaded region is
square centimeters, what is the area of the region that is not shaded?
A. sq cm
B. sq cm
C. sq cm
D. sq cm
E. sq cm
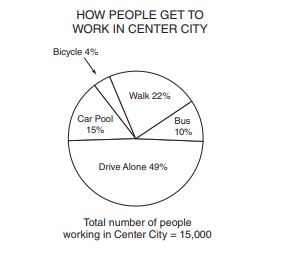
58.
How many more people in Center City walk to work than ride their bicycles to work?
F.
G.
H.
J.
K.
59. If and
are positive integers such that
, what is the least possible value for
?
A.
B.
C.
D.
E.
60.
In the equation above, what is the value of ?
F.
G.
H.
J.
K.
61. Ms. Grant’s car gets between and
miles per gallon, inclusive. The gasoline she uses costs between
and
per gallon, inclusive. What is the greatest amount Ms. Grant will spend on gasoline to drive her car
miles?
A.
B.
C.
D.
E.
62. The set consists of all prime numbers greater than
and less than
. What is the median of the numbers in
?
F.
G.
H.
J.
K.
63. What is the greatest common factor of and
?
A.
B.
C.
D.
E.
64.
.
.
.
______
If the missing terms in the problem above were filled in according to the pattern, what would be the sum of all the terms?
F.
G.
H.
J.
K.
65.
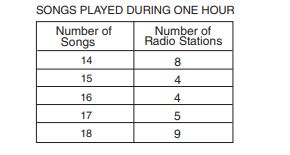
The table above shows the number of songs played during a specific hour by different radio stations. What is the mean number of songs played during that hour by these stations?
A.
B.
C.
D.
E.
66. The fuel mix for a small engine contains only ingredients: gasoline and oil. If the mix requires
ounces of gasoline for every
ounces of oil, how many ounces of gasoline are needed to make
ounces of fuel mix?
F.
G.
H.
J.
K.
67. In the set of consecutive integers from to
, inclusive, there are
integers that are multiples of both
and
. How many integers in the set are multiples of neither
nor
?
A.
B.
C.
D.
E.
68. A pitcher contained ounces of orange juice and
ounces of grapefruit juice. More grapefruit juice was added to the pitcher until grapefruit juice represented
of the pitcher’s contents. How many ounces of grapefruit juice were added?
F. oz
G. oz
H. oz
J. oz
K. oz
69. A roofing contractor uses shingles at a rate of bundles for each
square feet of roof covered. At this rate, how many bundles will he need to cover a roof that is
square feet?
A.
B.
C.
D.
E.
70. How many ways can the letters in the word RAIN be arranged horizontally so that the vowels (A and I) are always immediately next to each other (either AI or IA)?
F.
G.
H.
J.
K.
71.
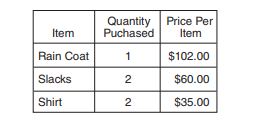
One state has a sales tax on clothing items priced at
or higher, and no sales tax on clothing items priced under
. What is the total tax on the items in the table above?
A.
B.
C.
D.
E.
72. There are eighth graders and
seventh graders in a school club. The president of this club wants
of the club’s members to be seventh graders. How many more seventh graders must join the club in order to meet the president’s wishes? (Assume that the number of eighth graders remains the same.)
F.
G.
H.
J.
K.
73. If and
are integers and
and
are both odd numbers, which of the following must be an even number?
A.
B.
C.
D.
E.
74. For what value of is
F.
G.
H.
J.
K.
75.
Nikolai bought a packet of pens. His receipt is shown above. Assume that sales tax is rounded to the nearest cent. If the sales tax had been computed on the sale price instead of on the regular price, how much lower would the tax have been?
A.
B.
C.
D.
E.
76. The regular price of a -ounce bag of candy is
. Lily has a coupon for
off one of these bags. What is the price per ounce (to the nearest cent) that Lily will pay if she uses the coupon?
F.
G.
H.
J.
K.
77. On a particular vehicle, the front tire makes three revolutions for every one revolution the back tire makes. How many times larger is the radius of the back tire than the radius of the front tire?
A.
B.
C.
D.
E.
78.
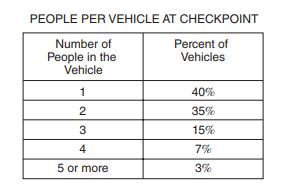
A researcher recorded the number of people in each vehicle that passed through a checkpoint. The table above shows the percent distribution for the vehicles that passed the checkpoint yesterday morning.
How many of the vehicles contained at least
people?
F.
G.
H.
J.
K.
79. Jack and Roberto were assigned to guard a tower. Each was to watch for hours, then rest
hours while the other watched. If Roberto began his first watch at 6:00 p.m., at what time will he begin his third watch?
A. 11:00 p.m.
B. 4:00 a.m.
C. 9:00 a.m.
D. 7:00 p.m.
E. 2:00 p.m.
80.
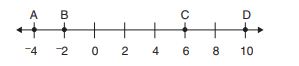
On the number line above, point (not shown) is the midpoint of
and point
(not shown) is the midpoint of
. What is the length of
?
F. unit
G. units
H. units
J. units
K. units
81. A video game originally priced at was on sale for
off. Julian received a
employee discount applied to the sale price. How much did Julian pay for the video game? (Assume that there is no tax.)
A.
B.
C.
D.
E.
82. If and
for
or
, what is the least possible value of
?
F.
G.
H.
J.
K.
83.
A.
B.
C.
D.
E.
84. There are cubic centimeters in
liter and
cubic millimeters in
milliliter. How many cubic millimeters are there in
cubic centimeters?
F.
G.
H.
J.
K.
85. A radio station plays Samantha’s favorite song times each day at random times between 8:00 a.m. and 5:00 p.m. The song is
minutes long. If Samantha turns on the radio at a random time between 8:00 a.m. and 5:00 p.m., what is the probability that her favorite song will be playing at that time?
A.
B.
C.
D.
E.
86. Set contains all integers from
to
, inclusive, and Set
contains all integers from
to
, inclusive. How many integers are included in
, but not in
?
F.
G.
H.
J.
K.
87. Ryan must read pages for school tomorrow. It took him
minutes to read the first
of the assigned pages. At this rate, how much additional time will it take him to finish the reading?
A. hrs
B. hrs
C. hrs
D. hrs
E. hrs
88.
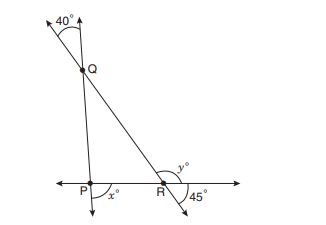
The figure above shows three intersecting straight lines. What is the value of ?
F.
G.
H.
J.
K.
89. Joe began to increase the speed of his car at 2:00 p.m. Since that time, the speed of Joe’s car has been steadily increasing by miles per hour for each half minute that has passed. If the car is now traveling
miles per hour, for how many minutes has the car been exceeding the speed limit of
miles per hour?
A. mins
B. mins
C. mins
D. mins
E. mins
90. If and
are numbers such that
, what is the value of
?
F.
G.
H.
J.
K.
91. Let .
What is the value of ?
A.
B.
C.
D.
E.
92.
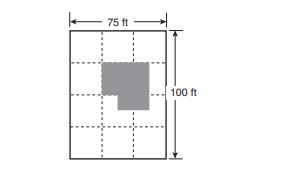
The drawing above represents a rectangular lot containing a building, indicated by the shaded region. The dashed lines divide the lot into twelve equal-sized squares. If the unshaded portion of the lot is to be paved, about how many square feet will be paved?
F. sq ft
G. sq ft
H. sq ft
J. sq ft
K. sq ft
93. If can be any integer, what is the greatest possible value of the expression
?
A.
B.
C.
D.
E. Infinity
94. A recent survey asked students what pets they have. Based on the results, the following statements are all true:
students have dogs.
students have cats.
students have both dogs and cats.
students have no cats or dogs.
How many students were surveyed?
F.
G.
H.
J.
K.
95. Ang has dollars in his savings account, and Julia has
dollars in her savings account. Ang gives Julia
of the money in his savings account, which Julia deposits into her savings account. Julia then spends
of the total in her savings account.
Express the amount of money Julia spent in terms of and
.
A.
B.
C.
D.
E.
96. Nam worked on a job for days. On each of the last
days, he worked
hours more than the mean number of hours he worked per day during the first
days. If he worked
hours in all, how many hours did he work during the last
days together?
F.
G.
H.
J.
K.
97.
The table above shows prices for newspaper advertising. A store purchased quarter pages, half pages, and full pages of space in equal numbers for a total of . What is the total amount of page space the store purchased?
A. pages
B. pages
C. pages
D. pages
E. pages
98. One week the price of gasoline dropped by per gallon. Madison’s car travels
miles each way to work, and her car travels
miles on each gallon of gasoline. What were her total savings, to the nearest cent, over the
-day work week?
F.
G.
H.
J.
K.
99. A rectangular floor is feet wide and
feet long. It must be covered with square tiles that are
inches on each side. Assume there is no space between adjacent tiles. If the tiles cost
each, how much will it cost to buy the tiles needed to cover the floor?
A.
B.
C.
D.
E.
100. {}
Company X wants to assign each employee a -digit ID number formed from digits in the set shown above. No digit may appear more than once in an ID number, and no two employees may be assigned the same ID number. What is the greatest total number of
possible different ID numbers?
F.
G.
H.
J.
K.
============================
51.
A.
B.
C.
D.
E.
52. Jack scored a mean of points per game in his first
basketball games. In his
th game, he scored
points. What was Jack’s mean score for the
games?
F.
G.
H.
J.
K.
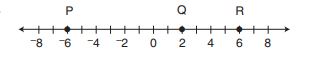
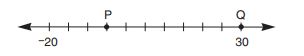
53.
How many units is it from the midpoint of to the midpoint of
?
A.
B.
C.
D.
E.
54. Each child in a certain class is required to have school supplies of notebook and
pencils. One notebook costs
and one pencil costs
. With
, what is the maximum number of children that can be provided with the required supplies?
(Assume no tax.)
F.
G.
H.
J.
K.
55. What time will it be hours after 9:30 p.m. on Friday?
A. 7:30 p.m. Saturday
B. 7:30 a.m. Sunday
C. 6:30 p.m. Sunday
D. 7:30 p.m. Sunday
E. 9:30 p.m. Sunday
56. Judy is years older than Carmen and twice as old as Frances. If Frances is
, how old is Carmen?
F.
G.
H.
J.
K.
57. If , what is the value of
?
A.
B.
C.
D.
E.
58. On a scale drawing, a distance of foot is represented by a segment
inch in length. How long must a segment on the scale drawing be to represent a
-inch distance?
F. in.
G. in.
H. in.
J. in.
K. in.
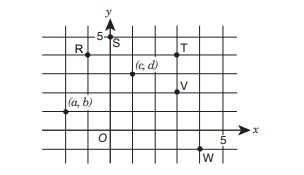
59.
The figure above is drawn to scale. Which point best shows the location of ?
A. R
B. S
C. T
D. V
E. W
60.
F.
G.
H.
J.
K.
61. dollar
lorgs
dollar
dalts
Kwamme has lorgs and
dalts. If he exchanges the lorgs and dalts for dollars according to the rates above, how many dollars will he receive? (Assume there are no exchange fees.)
A.
B.
C.
D.
E.
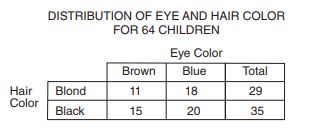
62.
The table above shows the distribution of eye color and hair color for children. How many of these children have blond hair or brown eyes, but not both?
F.
G.
H.
J.
K.
63. sind
ricks
sind
dalts
Using the conversions above, how many dalts are equivalent to rick?
A. dalts
B. dalts
C. dalts
D. dalts
E. dalts
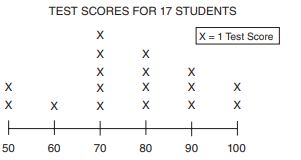
64.
According to the figure above, what was the median score for the test?
F.
G.
H.
J.
K.
65. What is the solution to
A.
B.
C.
D.
E.
66. Which of the following shows the fractions ,
, and
in order from least to greatest?
F. ,
,
G. ,
,
H. ,
,
J. ,
,
K. ,
,
67.
Point is to be placed on the number line one-third of the way from point
to point
. What number will be at the midpoint of segment
?
A.
B.
C.
D.
E.
68. A prom dress originally priced at is on sale for
off the original price. In addition,
Alia has a coupon for off the discounted price. If there is a
sales tax on the final price of the dress, what would Alia’s total cost be?
F.
G.
H.
J.
K.
69. How many different two-digit numbers can be formed from the digits if the numbers must be even and no digit can be repeated?
A.
B.
C.
D.
E.
70. A group of mountain climbers started the day at an elevation of feet below sea level. At the end of the day, they camped at
feet above sea level. What was the climbers’ elevation gain for the day?
F. ft
G. ft
H. ft
J. ft
K. ft
71. How many integers are between and
?
A.
B.
C.
D.
E.
72.
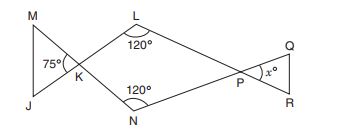
In the figure above, ,
,
, and
are straight segments. What is the value of
?
F.
G.
H.
J.
K.
73.
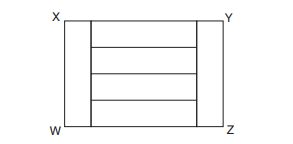
Figure above is composed of
congruent rectangular panels. The area of figure
is
square centimeters. What is the perimeter of figure
in centimeters?
A. cm
B. cm
C. cm
D. cm
E. cm
74. What is the prime factorization of ?
F.
G.
H.
J.
K.
75. Three gallons of gasoline are needed to drive miles. At this rate, how many gallons are needed to drive
miles?
A. gal.
B. gal.
C. gal.
D. gal.
E. gal.
76. If Crystal multiplies her age by and then adds
, she will get a number equal to her mother’s age. If
is her mother’s age, what is Crystal’s age in terms of
?
F.
G.
H.
J.
K.
77.
Points and
are points on the number line above, which is divided into equal sections. What is the value of
?
A.
B.
C.
D.
E.
78. 8:54 a.m.
9:12 a.m.
9:24 a.m.
10:24 a.m.
11:18 a.m.
Light A flashes every minutes, and light B flashes every
minutes. The two lights flash at the same time at 8:00 a.m. At how many of the times listed above will they again both flash at the same time?
F.
G.
H.
J.
K.
79. Which sum below can be expressed as a non-repeating decimal?
A.
B.
C.
D.
E.
80. To paint a room, Suzanne uses blue and white paint in the ratio of blue:white . What was the total number of gallons of paint used if she used
gallons of blue paint?
F. gal.
G. gal.
H. gal.
J. gal.
K. gal.
81. A cylindrical oil drum can hold 4,320 liters when it is completely full. Currently, the drum is full of oil. How many kiloliters of oil need to be added to fill the drum
completely?
A. kL.
B. kL.
C. kL.
D. kL.
E. kL.
82.
On the number line above, is located at
,
is located at
, and
is located at
.
(not shown) is the midpoint of
, and
(not shown) is the midpoint of
. What is the midpoint of
?
F.
G.
H.
J.
K.
83. A certain insect has a mass of milligrams. What is the insect’s mass in grams?
A. g
B. g
C. g
D. g
E. g
84. A box contains marbles —
red and
green. Five of these marbles are removed at random. If the probability of drawing a green marble is now
, how many red marbles were removed from the box?
F.
G.
H.
J.
K.
85. A water tank is full; then,
gallons of water is added to the tank, making it
full. How many gallons of water could the tank hold if it were completely full?
A. gal.
B. gal.
C. gal.
D. gal.
E. gal.
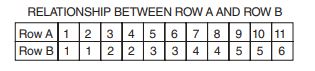
86.
The table above shows two rows of integers, Row A and Row B, and the relationship between them. Assume each row continues in the pattern shown. When the number appears in Row A, what is the corresponding number that will appear in Row B?
F.
G.
H.
J.
K.
87. In a restaurant, the mean annual salary of the chefs is
, and the mean annual salary of the
waiters is
. What is the mean annual salary of all
employees?
A.
B.
C.
D.
E.
88. On the first leg of its trip, a plane flew the 900 miles from New York City to Atlanta in 2 hours. On the second leg, it flew the 1,400 miles from Atlanta to Albuquerque in hours. How much greater was the plane’s mean speed, in miles per hour, on the second leg than on the first?
F. mph
G. mph
H. mph
J. mph
K. mph
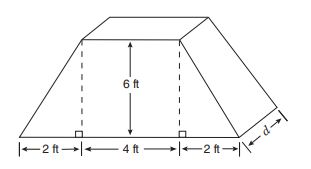
89.
The end of a tent has a trapezoidal cross-section as shown above. What is the depth of the tent if its volume is
cubic feet?
A. ft
B. ft
C. ft
D. ft
E. ft
90. Today, Tom is of Jordan’s age. In
years, Tom will be
of Jordan’s age. How old is Jordan today?
F.
G.
H.
J.
K.
91. How many positive two-digit numbers are evenly divisible by ?
A.
B.
C.
D.
E.
92. A steel container is shaped like a cube feet on each side. This container is being filled with water at a rate of
cubic feet per minute. At the same time, water is leaking from the bottom of the container at a rate of
cubic feet per minute. If the container is exactly half-filled at 9:00 a.m., at what time will the container begin to overflow?
F. 9:55 a.m.
G. 10:00 a.m.
H. 10:11 a.m.
J. 10:40 a.m.
K. 12:20 p.m.
93. Each week, Arnold has fixed expenses of at his furniture shop. It costs Arnold
to make a chair in his shop, and he sells each chair for
. What is Arnold’s profit if he makes and sells
chairs in
week?
A.
B.
C.
D.
E.
94. In how many different ways can you make exactly using only nickels, dimes, and quarters, if you must have at least one of each coin?
F.
G.
H.
J.
K.
95.
A.
B.
C.
D.
E.
96. A -gram mixture contains three items, X, Y, and Z. The ratio of the weights of X and Y is
, and the ratio of the weights of Y and Z is
. If all of item Z were removed, what would be the new weight of the mixture?
F. g
G. g
H. g
J. g
K. g
97. Maria is now years old. In
years, she will be twice as old as her brother is then. How old is her brother now?
A.
B.
C.
D.
E.
98. A car travels at 4,400 feet per minute. If the radius of each tire on the car is one foot, how many revolutions does one of these tires make in a single minute? (Use the approximation for
.)
F.
G.
H.
J.
K.
99. Which number line below shows the solution to the inequality ?
A.
B.
C.
D.
E.
100. What is the greatest prime factor of ?
F.
G.
H.
J.
K.
=====================================================================
Grade 9 Math
1.In the figure above, all lines are straight. and
intersect at point
. What is the value of
?
A.
B.
C.
D.
E.
2. The translation of point to
is
equivalent to rotating point by which of the following clockwise rotations about the origin?
F.
G.
H.
J.
K.
3.
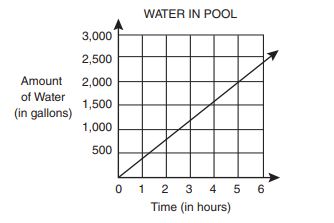
A swimming pool is being filled with water at a constant rate. The figure above is a portion of a graph that shows how the number of gallons of water in the pool changes over time.
Starting with an empty pool, at the end of hour there are
gallons in the pool. If the pool continues to fill at this rate, how much water will be in the pool at the end of hour
? (Assume that the pool holds a total of
gallons.)
A. gal.
B. gal.
C. gal.
D. gal.
E. gal.
4. If , what is the value of
?
F.
G.
H.
J.
K.
5.
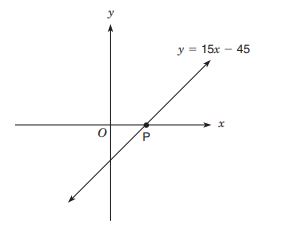
The line defined by the equation intercepts the
-axis at point
as shown above. What are the coordinates of point
?
A.
B.
C.
D.
E.
6.
On the number line above, which letter could represent the location of ?
F. R
G. S
H. T
J. U
K. V
7. If , what is the value of
?
A.
B.
C.
D.
E.
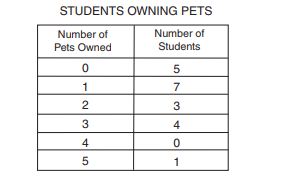
8.
There are students in a class. The frequency table above shows the number of these students that own
or
pets. What is the mean number of pets owned per student in this class?
F.
G.
H.
J.
K.
9. The temperature inside an oven when it is off is F. When Gail turns the oven on, it heats at a constant rate, reaching a temperature of
F in
minutes. Which equation indicates the temperature
of the oven
minutes after it is turned on?
A.
B.
C.
D.
E.
10. ,
,
How many integer values of satisfy both inequalities shown above?
F.
G.
H.
J.
K.
11. ,
,
,
,
If , which one of the expressions above does not represent a rational number?
A.
B.
C.
D.
E.
12. Let . Using that rule, if
what is
?
F.
G.
H.
J.
K.
13. Seven consecutive integers are arranged in increasing order. Their sum is . What is the value of the second integer in terms of
?
A.
B.
C.
D.
E.
14. Define the operation as follows:
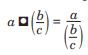
and
are not zero.
If , what is the value of
?
F.
G.
H.
J.
K.
15. Raul has two containers. One is a cylinder with an inner radius of inches and an inner height of
inches. The other is a cube with inner height, width, and length each equal to
inches. The cylinder is filled with water and the cube is empty. If Raul pours the contents of the cylinder into the cube, how deep will the water be in the cube?
A. in.
B. in.
C. in.
D. in.
E. in.
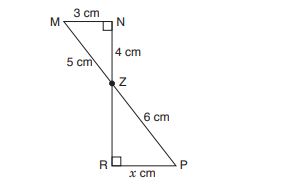
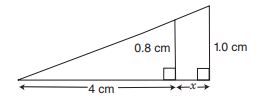
16.
In the figure above, what is the value of ?
F. cm
G. cm
H. cm
J. cm
K. cm
17. Straight line passes through the point
with an
-intercept of
. What is the equation of line
?
A.
B.
C.
D.
E.
Additional SHSAT Resources
Online SHSAT Tutoring
Online SHSAT Prep
Free SHSAT Practice Test
Preparing for the SHSAT and TACHS at the Same Time
SHSAT Cut-Off Scores
SHSAT 2015-2016
SHSAT 2016-2017