Writing Scientific Notation

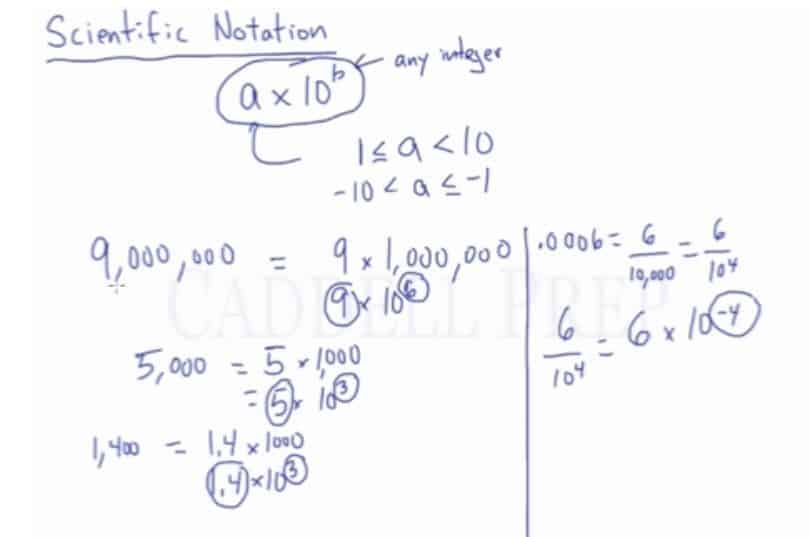
Examples of Writing Scientific Notation

Example 1

0.000016

First, let’s get a number between 1 and 10

That is 1.6

Then, let’s count the decimal spaces to have the value of our exponent

We counted 5 decimal spaces

So we’ll have

0.000016= 1.6 \times 10^5

Since, we counted from left to right. We have to counteract that by making the value of our exponent negative

Therefore, our final answer is

0.000016= 1.6 \times 10^{-5}

Example 2

23,400,000

First, let’s get a number between 1 and 10

That is 2.34

Then, let’s count how many decimal spaces are there in 23,400,000 for us to get 2.34

There are 7 decimal spaces.

Since, we counted from the right to left. Meaning it’s a big number and we’re making it small.

Now, we have:

23,400,000= 2.34\times 10^7

Video-Lesson Transcript

In this lesson, you will learn how to write numbers in scientific notation. After you finish this lesson, view all of our Pre-Algebra lessons and practice problems.

The basic form of scientific notation is a \times 10^b

Whereas b can be any integer and can be positive or negative.

Whereas a is 1 \leq a \textless 10

Or a can also be negative -10 \textless a \leq -1

Whereas 10 is constant.

Here’s an example:

9,000,000 = 9 \times 1,000,000

It’s really = 9 \times 10^6

Let’s say we have 5,000

It’s really = 5 \times 1,000

and then = 5 \times 10^3

If we have 1,400

it could be written as = 1.4 \times 1,000

which really is = 1.4 \times 10^3

See 9, 5, and 1.4 are our a. All of them meet the requirement above.

Likewise, all the exponents are integers.

Here’s an example of a negative exponent.

0.0006

This is = \dfrac{6}{10,000}

which is = \dfrac{6}{(10^4)}

To review our lesson in Exponents, we have to change the sign to move the denominator to the numerator.

So since we want to move the denominator to the numerator, we have to write = 6 \times 10^{-4}

Write Numbers In Scientific Notation

This is a bit tedious work.

Don’t worry. There’s a quicker way to do this!

Writing Scientific Notation by Counting Decimal Places

For example, we have 3,000

Let’s get a where 1 \leq a \textless 10 then multiplied by 10

So it’s = 3 \times 10

Then let’s count the decimal spaces from the right of 3,000 and stop to get 3.

We counted 3 decimal spaces.

This goes right in as our exponent.

So now we have 3,000 = 3 \times 10^3

Let’s have another one.

54,000

Remember, we need to have a number that is less than 10 so we can’t have 54.

Instead, we can have 5.4

So, we’ll have 5.4 \times 10

Then let’s count how many decimal spaces are there in 54,000 for us to get 5.4

There are 4 decimal spaces. This goes right in as our exponent.

We counted from the right to left. Meaning it’s a big number and we’re making it small.

Now, we have 54,000 = 5.4 \times 10^4

Now, let’s have a small number.

0.0002

Let’s get a number between 1 and 10.

That is 2

Multiplied by 10 which is constant

Then let’s count the decimal spaces to have the value of our exponent.

We counted 4 decimal spaces.

So we’ll have 0.0002 = 2 \times 10^4

But remember we counted from left to right. Which means it’s a small number and we’re making it big number.

We have to counteract that by making the value of our exponent negative.

So our final answer is 0.0002 = 2 \times 10^{-4}

Exponent should be in negative if we have a small number and we’re writing it to appear a big number.

On the other hand, an exponent is positive if our original number is big and we’re writing it to become a small number.

Let’s have one more example.

We have 0.0056

The number between 1 and 10 is 5.6

Our exponent is 3 since we moved three decimal spaces to get 5.6.

Moreover, it should be in negative because we have a small number.

So the scientific notation is 0.0056 = 5.6 \times 10^{-3}

Another way to look at the value of exponent:

If you moved the decimal to the left, you’ll have a positive exponent.

And if you moved the decimal to the right, you’ll have a negative exponent.