Solving A System Of Equations Using Substitution (Linear & Quadratic)

In this video, we are going to look at how to solve a system of equations using substitution – linear and quadratic. These are solved similarly to how we would normally solve any other system of equations. After you finish this lesson, view all of our Algebra 1 lessons and practice problems.

For example:


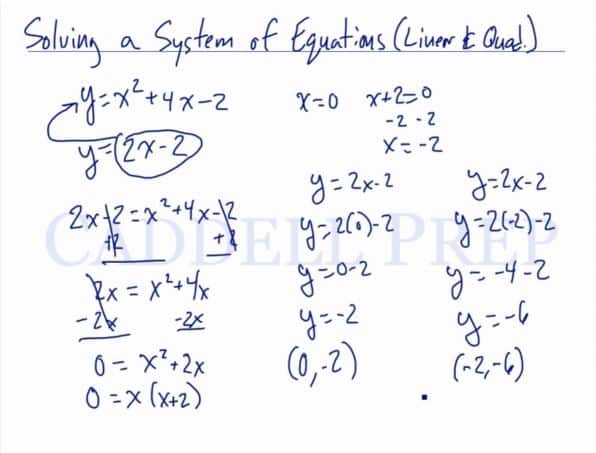
To solve, we are going to take , which equals and substitute it into the first equation where we see . Now we have

Since it is a quadratic, we want to get one side equal to 0 so we can factor. First, add 2 to both sides to get

Then subtract 2x from both sides to get

When we factor, we get

Set each factor equal to 0
and
We find that

Now to get the y values, we substitute these x values into an equation. If we substitute them into then we have
which simplifies to
which simplifies to
Therefore, (0,-2) and (-2,-6) are the solutions to this system of equations.

Example of Solving A System Of Equations Using Substitution (Linear & Quadratic)

Example 1

Let’s substitute the first equation as the value of in the second equation.

Get one side to be zero.

Now, let’s factor this.

Then

and

Now, let’s substitute the values of to get the value of .
Let’s use the first equation.
In ,

In ,

So our answers are:
{}

Example 2

Let’s substitute the first equation as the value of in the second equation.

Get one side to be zero.

Now, let’s factor this.

Then

and

Now, let’s substitute the values of to get the value of .
Let’s use the first equation.
In ,

In ,

So our answers are:
{}

Video-Lesson Transcript

Let’s go over solving a system of equations algebraically for linear and quadratic functions.

This is very similar to how you solve a system of linear equations except we have a variable raised to the second power.

For example:

Now, let’s substitute one expression to the value of the other equation.

In this case, let’s get the second equation and substitute it in the first equation.

Now, we have

Like normal, we’ll have one side of it to be equal zero.

There’s no -term so we’re going to factor this.

Identify the greatest common factor

Now, we have two factors which is each equal to zero.

and

Now, let’s solve for the value of by substituting the value of in one of the equations.

In ,

Here, our answer is .

In ,

Here, our answer is

Let’s try another system of equations.

Same thing, let’s substitute the first equation as the value of in the second equation.

Same strategy. Get one side to be zero.

Now, let’s factor this.

Then

and

Now, let’s substitute the values of to get the value of .

Let’s use the first equation.

In ,

In ,

So our answers are:

{}