Caddell Prep Access Menu

Navigation

Simplifying Radical Expressions

In this video, we are going to simplify radical expressions. Note that a perfect square number has a rational square root.

  • Join today & get access to 1,000's of practice problems

\sqrt{4}=2 because 2^2=4,
\sqrt{9}=3 because 3^2=9,
\sqrt{16}=4 because 4^2=16, and so on…

There are other radical expressions other than perfect square ones, to which they may not have a rational square root.
\sqrt{5} and \sqrt{20}, and \sqrt{32} are some examples.

However, having an irrational square doesn’t mean that the radical expression cannot be simplified.
\sqrt{5} cannot be simplified because it is in its simplest state.

For \sqrt{20}, 4 and 5 are factors of 20. Choosing factors that are perfect squares make the simplifying process easier.
\sqrt{20} = \sqrt{4}\times\sqrt{5}
\sqrt{4} is 2, so \sqrt{4}\times\sqrt{5} is really just 2\sqrt{5}

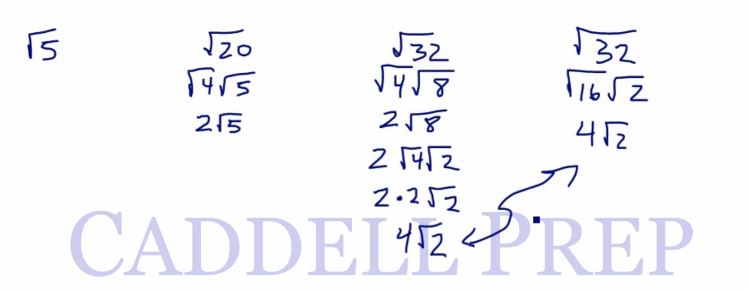
For \sqrt{32}, 4 and 8 are factors of 32.
\sqrt{32} = \sqrt{4}\times\sqrt{8}
\sqrt{4} is 2, so \sqrt{4}\times\sqrt{8} is 2\sqrt{8}
\sqrt{8} can be further simplified into \sqrt{4} and \sqrt{2}, or 2\times\sqrt{2}
In the end, \sqrt{32} is 4\sqrt{2}

Another way of simplifying \sqrt{32} is to use \sqrt{16} and \sqrt{2} as the factors.
Since 16 is a perfect square, \sqrt{16}\times\sqrt{2} can be written as 4\sqrt{2}, leading to the answer immediately.

Simplifying Radical Expressions

Now let’s try three more examples:
\sqrt{75}
25 is a perfect square that goes into 75 and the other factor is 3
The square root of 25 is 5 so the final answer is 5\sqrt{3}

\sqrt{48}
4 and 12 go into 48
\sqrt{4} can be broken down into 2 and \sqrt{12} can be broken down into \sqrt{4} and \sqrt{3}
The square root of 4 is 2, 2\times2\times\sqrt{3}, so we have 4\sqrt{3}

Now let’s do the same problem by using the perfect square
16 is a perfect of 48 and the accompanying factor is 3
The square root of 16 is 4, so we have 4\sqrt{3}, same answer

\sqrt{63}
9 is a perfect square that goes into 63 and the other factor is 7
The square root of 9 is 3 so the final answer is 3\sqrt{7}

Video-Lesson Transcript

Let’s go over how to simplify radical expressions.

Let’s start with some basic ones.

\sqrt{4} = 2 because 2^2 = 4; exponent is the inverse of square root

\sqrt{9} = 3
\sqrt{16} = 4
\sqrt{25} = 5
\sqrt{36} = 6
\sqrt{49} = 7
\sqrt{64} = 8
\sqrt{81} = 9
\sqrt{100} = 10

These are all known as perfect squares.

The rational answer exists.

These are rational expressions. But sometimes we have the square root of a number that we can’t do the square root of. That is called an irrational.

For example:

\sqrt{5}

There is no integer that you can multiply by itself that will give you 5.

Other examples of irrational are

\sqrt{20}
\sqrt{32}

But even if they are irrational, doesn’t mean that we cannot reduce them.

So let’s take a look at that next.

\sqrt{5} cannot be reduced

Next, \sqrt{20}.

What numbers go into 20?

Well, 4 and 5.

We want to pick these numbers since 4 is a perfect square.

So it will be

\sqrt{20}
\sqrt{4} \sqrt{5}
2 \sqrt{5}

Now, let’s see

\sqrt{32}
\sqrt{4} \sqrt{8}
2 \sqrt{8}
2 \sqrt{4} \sqrt{2}
2 \times 2 \sqrt{2}
4 \sqrt{2}

Now, let’s think about this.

We were able to break \sqrt{32} twice.

First into 4 and 8. Then 8 into 4 and 2.

Probably there’s a bigger number that we could’ve picked that goes into \sqrt{32}.

That is 16.

So let’s do it again.

\sqrt{32}
\sqrt{16} \sqrt{2}
4 \sqrt{2}

And we came up with the same answer.

Notice that if we start off with the biggest possible perfect square that goes into a number, we’ll get the answer immediately.

If we don’t, like what we did prior to this, we’ll get the same answer but took us some more steps.

Let’s have three more examples.

\sqrt{75}

What perfect square goes into 75?

25 does.

\sqrt{75}
\sqrt{25} \sqrt{3}
5 \sqrt{3}

Now, \sqrt{48}.

What’s the largest perfect square that goes into 48?

Let’s try this

\sqrt{48}
\sqrt{4} \sqrt{12}
2 \sqrt{12}
2 \sqrt{4} \sqrt{3}
2 \times 2 \sqrt{3}
4 \sqrt{3}

As you can see, we break this down twice.

So we probably didn’t get the biggest perfect square in the beginning.

Let’s do it again.

\sqrt{48}
\sqrt{16} \sqrt{3}
4 \sqrt{3}

We still got the same answer as above.

Now, let’s look at \sqrt{63}

What goes into 63 and is a perfect square?

\sqrt{63}
\sqrt{9} \sqrt{7}

So, our answer is

3 \sqrt{7}