Learn The Properties of Exponential Growth And Its Functions

In this video, we are going to look at exponential growth. After you finish this lesson, view all of our Algebra 1 lessons and practice problems.

$y=a \times (b)^x$
For a graph to demonstrate exponential growth, the coefficient, a, must be positive, and the base of the exponent, b, must be greater than 1. The graph will follow a path that curves upward to the right. These graphs grow by a factor, as opposed to a constant value.

For example:

The graph of
$y=2 \times (1.2)^x$
curves upward after plotting points as shown in the video, and demonstrates exponential growth. This graph grows by a factor of 1.2.

When comparing a linear graph to an exponential growth graph, as more time goes on, the exponential growth tends to grow by larger quantities than the linear growth.

Examples of Exponential Growth

Example 1

The population of Greendale in $2016$ was estimated to be $35,000$ people with an annual rate of increase of $2.4%$ . What will be the estimated population in year 2020?

After one year the population would be $35,000 + 0.024(35,000)$ .
By factoring, we have $35,000(1 + 0.024)$ or $35,000(1.024)$ .

In year $2016$ or initally, the population is $35,000$ .

Then in year $2017$ , we have $35,840$ ,
year $2018$ , we have $36,700$ ,
in year $2019$ , we have $37,580$ ,
and in year $2020$ , we have $38,482$

Therefore, the estimated population in the year $2020$ is $38,482$ .

Example 2

If we have $\$50,000$ deposited in the bank, and receive a $2\%$ interest annually. How much is the money in the $3$ rd year?

After one year the money will be $50,000 + 0.02(50,000)$ .
By factoring, we have $50,000(1 + 0.02)$ or $50,000(1.02)$ .

In year $0$ or initally, the money deposited is $50,000$ .

Then in $1$ st year, we have $\$51,000$ ,
$2$ nd year, we have $\$52,020$ ,
and in the $3$ rd year, we have $\$53,060.40$

Therefore, the money will be $\$53,060.40$ in $3$ rd year.

Video-Lesson Transcript

Let’s go over exponential growth.

The basic exponential function is

$y = a \times b^x$ $y = ab^x$

In exponential growth, $a$ is always positive and $b \textgreater 1$ .

If we look at the exponential growth graph, it will look like this.

Where each point is some fact greater than the next one.

For example:

$b = 1.2$
$a = 2$

then $y = 2 (1.2)^x$

Let’s see what this table looks like.

Let’s get a financial calculator to solve this.

So when $x = 0$ , $y = 2$ ,
when $x = 1$ , $y = 2.4$ ,
and when $x = 2$ , $y = 2.88$ .

So the question is how do we get to these points?

Each time we’re just multiplying by $b = 1.2$ .

So $2 \times 1.2 = 2.4$ , then $2.4 \times 1.2 = 2.88$ , and then $2.88 \times 1.2 = 3.456$ .

The next point is $x = 3$ .

Each time we multiply by $b = 1.2$ . It multiplies by the constant $b$ .

It’s not going up by a said amount. Here, the increase in $y$ value is increasing in itself.

Whereas when we have a linear graph, it goes up at a constant rate each time.

Here, the jump in $y$ values are linear. The increase or decrease is the same in the entire time.

Just to recap, exponential growth has $x$ as an exponent for it to be exponential, growth is a positive number greater than $1$ .

Very important:

Exponential growth grows by a factor.

So you keep on multiplying by a certain number to get a new value.

An example is a compound interest. Each month money may grow by $8\%$ or some value compared to the previous one.

Whereas, linear growth grows by a constant value.

So instead of growing by a certain percentage, it will always grow by a certain amount.

For example, your money grows by $\$20$ each month.

On the other hand, exponential growth may grow your money depending on the percentage.

Let’s look at an example:

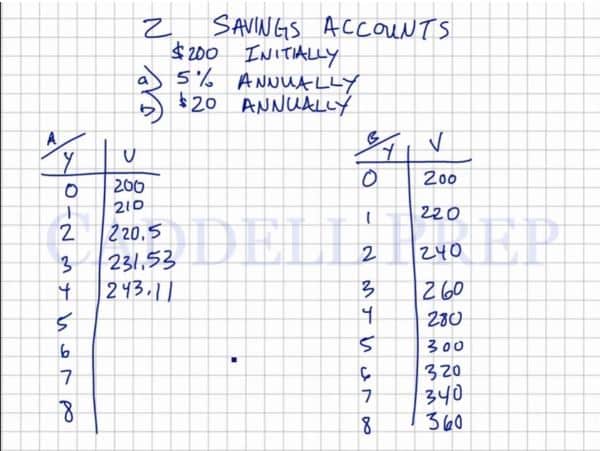
Two Savings Accounts
$\$200$ initially

a) $5\%$ annually
b) $\$20$ annually

Let’s start with savings account b where money grows by $\$20$ annually.

Year $0$ or initally, we have $\$200$ .

Then year $1$ , we have $220$ ,
year $2$ , we have $240$ ,
on year $3$ , we have $260$ ,
year $4$ , we have $280$ ,
then year $5$ , we have $300$ ,
year $6$ , we have $320$ ,
year $7$ , we have $340$ ,
and year $8$ , we have $360$ .

Now, let’s do savings account a where it grows $5\%$ annually.

Year $0$ or initally, we have $\$200$ .

Then year $1$ , we have $210$ ,
year $2$ , we have $220.50$ ,
year $3$ , we have $231.53$ ,
and on year $4$ , we have $243.11$ .

Take note that the previous amount grows by $5\%$ . So we add the increase to the previous amount to get the new value.

Savings account b still looks further ahead.

Let’s make an equation for this.

$y = 200 (1.05)^x$

Let’s compare it to the one that grows $\$20$ every year.

So far, we have no increase of more than $\$20$ .

From year $10$ to $11$ , we have a $\$15$ growth. But still not there yet.

After $14$ years, we have an $\$18$ growth.

At year $16$ , the difference is $\$21$ .

From year $21$ to $22$ , it grew by almost $\$30$ .

From year $33$ to $34$ , it grew by $\$50$ .

It grows slower, depending on the numbers you’re comparing it to. But after a said amount of time, it will grow at a much greater rate. And will eventually pass the other one.

What if we have a $8\%$ increase?

So right off the bat, it improved by $\$16$ .

Then at year $4$ , it grew by more than $\$20$ .

From year $9$ to $10$ , it improved by $\$32$ .

The compound interest or exponential growth will quickly pass the linear growth.