Dividing Radical Expressions

In this video, we are going to divide radical expressions. After you finish this lesson, view all of our Algebra 1 lessons and practice problems.

One simple example is:
\dfrac{\sqrt{25}}{\sqrt{9}}
By simplifying the numerator and the denominator, we now have \dfrac{5}{3}

A slightly more difficult problem would be:
\dfrac{\sqrt{50}}{\sqrt{18}}
Just like the other problem, first find perfect square factors for each expression
\dfrac{\sqrt{25}\times\sqrt{2}}{\sqrt{9}\times\sqrt{2}}
Simplify each expression
\dfrac{5\sqrt{2}}{3\sqrt{2}}
And the final answer is \dfrac{5}{3}

If the expression has a radical in the denominator and a rational number in the numerator, then it is necessary to rationalize the fraction.
For example:
\dfrac{3}{\sqrt{10}}
Multiple the denominator to both the numerator and the denominator. Like terms cancel each other out so the final answer is:
\dfrac{3\times\sqrt{10}}{\sqrt{10}\times\sqrt{10}}
\dfrac{3\sqrt{10}}{10}

Dividing Radical Expressions

Examples of Dividing Radical Expressions

Example 1

\dfrac{3}{8\sqrt{2}}
Since the denominator has a radical, we have to rationalize the fraction.
Multiply the numerator and denominator by \sqrt{2}
\dfrac{3}{8\sqrt{2}}\cdot\dfrac{\sqrt{2}}{\sqrt{2}}
Now, we have
\dfrac{3\sqrt{2}}{8(2)}
The final answer is
\dfrac{3\sqrt{2}}{16}

Example 2

\dfrac{3xy^2}{\sqrt{25x^3y}}
Simplify first the terms inside the radical
\dfrac{3y}{\sqrt{25x^2}}
Then \sqrt{25} is 5 and \sqrt{x^2} is x
Now, we have
\dfrac{\sqrt{3y}}{5x}

Video-Lesson Transcript

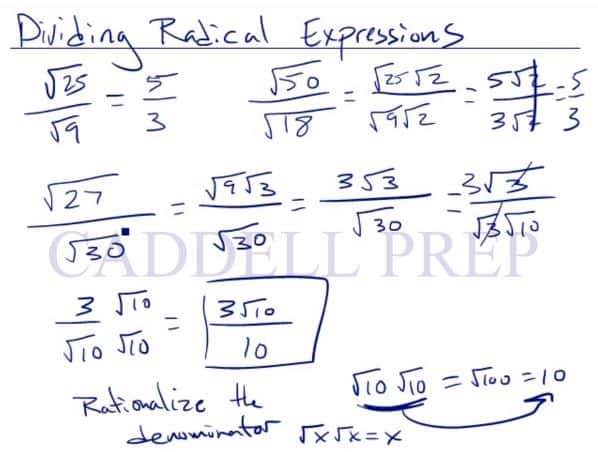
Let’s go over how to divide radical expressions.

Let’s say we have

\dfrac{\sqrt{25}}{\sqrt{9}}
\dfrac{5}{3}

And there’s not much to do.

But what if we have

\dfrac{\sqrt{50}}{\sqrt{18}}
= \dfrac{\sqrt{25} \sqrt{2}}{\sqrt{9} \sqrt {2}}
= \dfrac{5 \sqrt{2}}{3 \sqrt{2}}

And just like if we have

\dfrac{5x}{3x}

x will be cancelled out.

In this case, \sqrt{2} will be cancelled out.

So our answer is

= \dfrac{5}{3}

Of course, not all is going to work out like this.

Let’s look at another example.

\dfrac{\sqrt{27}}{\sqrt{30}}

Now, let’s break this down.

= \dfrac{\sqrt{9} \sqrt{3}}{\sqrt{30}}
= \dfrac{3 \sqrt{3}}{\sqrt{30}}

This is how far we can go as far as the radicals are concerned.

But this is not our final answer.

Because we don’t want a radical in the denominator.

But even more so, we can reduce this.

Just like this example:

\dfrac{\sqrt{15}}{\sqrt{5}}
= \dfrac{\sqrt{3}}{\sqrt{1}}
= \sqrt{3}

Another way to look at that is:

\dfrac{\sqrt{15}}{\sqrt{5}}
= \dfrac{\sqrt{5} \sqrt{3}}{\sqrt{5}}

\sqrt{5} cancels out and we’re left with

= \sqrt{3}

Now, let’s apply this in solving. Let’s go back

= \dfrac{3 \sqrt{3}}{\sqrt{30}}

We can break this down even more into

= \dfrac{3 \sqrt{3}}{\sqrt{3} \sqrt{10}}

Here, = \sqrt{3} is going to cancel out.

And we have

= \dfrac{3}{\sqrt{10}}

Now, we want to rationalize the denominator.

The trick in getting rid of \sqrt{10} is to multiply the numerator and denominator by the same number.

= \dfrac{3 \times \sqrt{10}}{\sqrt{10} \times \sqrt{10}}

This is our final answer

= \dfrac{3 \sqrt{10}}{10}

Now, let’s look at some more.

= \dfrac{ \sqrt{45}}{\sqrt{15}}

Let’s break this down

= \dfrac{\sqrt{9} \sqrt{5}}{\sqrt{15}}
= \dfrac{3 \sqrt{5}}{\sqrt{15}}

Then let’s reduce 5 and 15

We’ll have

= \dfrac{3 \sqrt{1}}{\sqrt{3}}
= \dfrac{3}{\sqrt{3}}

Now, we don’t want a radical in the denominator.

We have to rationalize it. So let’s multiply the numerator and denominator by \sqrt{3}.

= \dfrac{3 \times \sqrt{3}}{\sqrt{3} \times \sqrt{3}}
= \dfrac{3 \sqrt{3}}{3}

Which we cancel and we’re left with our final answer

= \sqrt{3}

This is a 100\% correct way to solve it.

Any way you solve for the correct answer is a good way to do it.

Let me show you another way.

We can reduce this right off the bat.

= \dfrac{ \sqrt{45}}{\sqrt{15}}

15 goes into 45, so this can be reduced to

= \dfrac{ \sqrt{3}}{\sqrt{1}}
\sqrt{3}

Two different options to solve the same problem. We have the same answer both ways.