Absolute Value Equations

In this video, we will be learning how to solve absolute value equations. After you finish this lesson, view all of our Algebra 1 lessons and practice problems.

***Remember*** Absolute Value is always Positive! (or zero) Therefore, some equations may have No Solution

For Example:

First subtract 3 to isolate the quantities in the absolute value brackets

Now remove the absolute value brackets and separate the equation into 2 cases as shown below

                                   

               

                                         

Examples of Absolute Value Equations

Example 1

First subtract 2 to isolate the quantities in the absolute value brackets

Now remove the absolute value brackets and separate the equation into 2 cases

                                   

                     

                                          

                                         

                                            

Example 2

First add 2 to isolate the quantities in the absolute value brackets

Now remove the absolute value brackets and separate the equation into 2 cases

                                   

                     

                                          

                                         

                                            

Video-Lesson Transcript

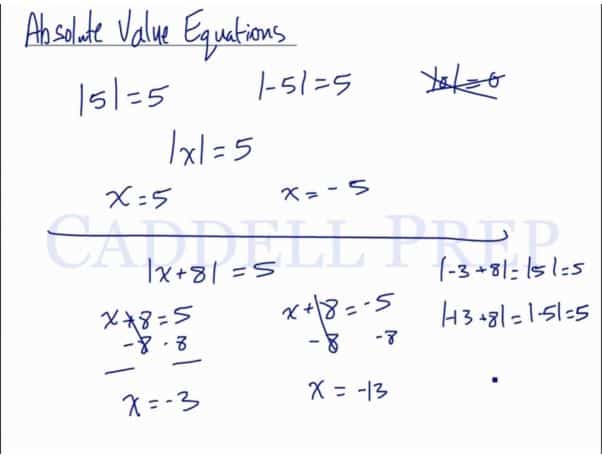
Let’s go over absolute value equations.

The absolute value is the distance of a number from .

The absolute value is the positive of that number.

For example:

Likewise

No matter what number we put in the absolute value, the answer is always positive.

Even if it’s zero. Zero is neither positive nor negative.

If , we can have two values for .

We can have or

Based on this concept, take a look at this:

Here, we can have two values of .

This makes two equations.

Because as we know earlier, or

First, let’s solve the left equation.

Let’s go to the other equation.

If we substitute these two values into , we’ll know they are both true.

Let’s do it!

Using ,

And using

So we already proved that both answers are correct.

Let’s take a look at another example.

Before we get our two equations, we have to get the absolute value by itself.

Now, let’s get rid of by subtracting it on both sides.

And

Another keypoint is there are some equations that has no solution.

For example:

Here, it’s impossible to get the value of to end up with a negative answer.

Because the absolute value of anything is always positive or zero.

Let’s have one more example.

In order to solve this, we have to have by itself.

Let’s solve now by subtracting on both sides.