Learn How To Solve Rational Equations

Solving Rational Equations by Getting LCD

In the first video, we are going to solve rational equations by getting the LCD (Least Common Denominator). After you finish this lesson, view all of our Algebra 1 lessons and practice problems.

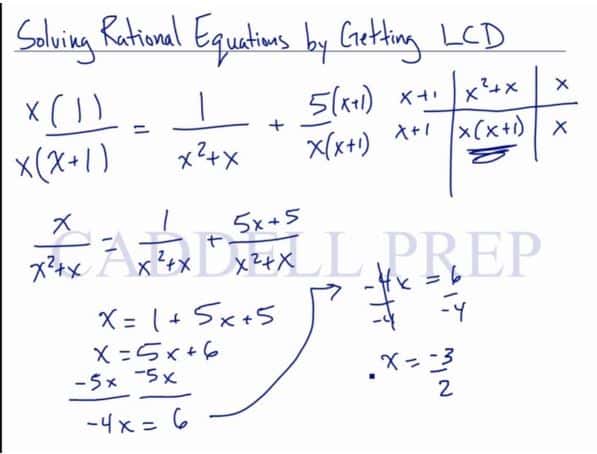
For Example: $\dfrac{1}{x+1}=\dfrac{1}{x^2+x}+\dfrac{5}{x}$

Find the LCD, which is $x^2+x$ . Multiple each expression to get its denominator to $x^2+x$
$\dfrac{(x)1}{(x)x+1}=\dfrac{1}{x^2+x}+\dfrac{(x+1)5}{(x+1)x}$

Multiply
$\dfrac{x}{x^2+x}=\dfrac{1}{x^2+x}+\dfrac{5x+5}{x^2+x}$

Now that the denominators are the same, we can add across
$x=1+5x+5$

Solve the equation for the value of x. Combine like terms
$x=5x+6$

Move x to one side of the equation
$-4x=+6$

Isolate x and we have
$x=-\dfrac{3}{2}$

Video-Lesson Transcript

Let’s go over how to solve rational equations.

In this method, we’re going to do it by getting the least common denominator (LCD).

$\dfrac{1}{x + 1} = \dfrac{1}{x^2 + x} + \dfrac{5}{x}$

First, let’s examine the denominators here.

One way to get the least common denominator is to multiply them together.

We want to examine these denominators so we can tell the factors.

$x + 1$ stays the same as well as $x$ .

But $x^2 + x$ can be factored out into $x (x + 1)$ .

This denominator is the first one times the last one.

Which makes this the least common denominator.

So, we’ll have

$\dfrac{x (1)}{x (x + 1)} = \dfrac{1}{x^2 + x} + \dfrac{5 (x + 1)}{x (x + 1)}$

$\dfrac{x}{x^2 + x} = \dfrac{1}{x^2 + x} + \dfrac{5x + 5}{x^2 + x}$

At this point, we can drop the denominators.

Since we already have common denominators, we can just add the numerators.

So, what’s really important is that we have a common denominator so we can add the numerators.

$x = 1 + 5x + 5$

Here, we can find the value of $x$ .

$x = 5x + 6$

$x - 5x = 5x - 5x + 6$

$-4x = 6$

$\dfrac{-4x}{-4} = \dfrac{6}{-4}$

$x = -\dfrac{3}{2}$

Solving Rational Equations by Multiplying by LCD

In the second video, we are going to solve rational equations by multiplying by the LCD (Least Common Denominator).

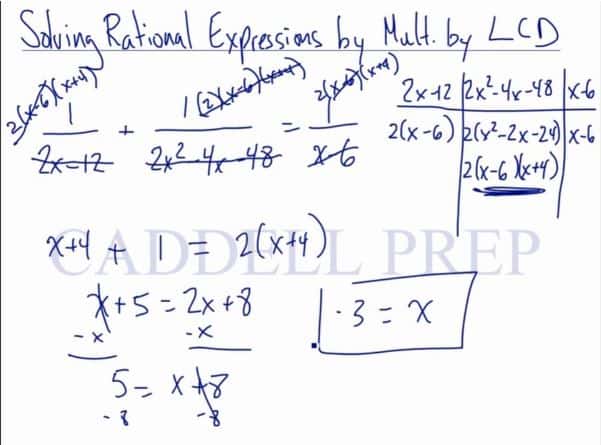
For example: $\frac{1}{2x-12}+\frac{1}{2x^2-4x-48}=\frac{1}{x-6}$

Determine the LCD of the three denominators, which is 2(x-6)(x+4). Multiply the numerators by the LCD
$\frac{2(x-6)(x+4)1}{2x-12}+\frac{2(x-6)(x+4)1}{2x^2-4x-48}=\frac{2(x-6)(x+4)1}{x-6}$

Reduce each expression
$\frac{(x+4)1}{1}+\frac{1}{1}=\frac{2(x+4)1}{1}$

Multiply
$x+4+1=2(x+4)$

Use the distributive property
$x+4+1=2x+8$

Combine like terms
$x+5=2x+8$

Move x to one side of the equation by subtracting x
$5=x+8$

Subtract 8 from both sides to isolate x, and we have
$-3=x$

Video-Lesson Transcript

Let’s go over solving rational expressions by multiplying by the least common denominator.

For example:

$\dfrac{1}{2x - 12} + \dfrac{1}{2x^2 - 4x - 48} = \dfrac{1}{x - 6}$

First, let’s look at the denominators.

$2x - 12$

$2x^2 - 4x - 48$

$x - 6$

We’ll get the common denominator by multiplying these together.

For us to get the least common denominator, let’s factor and see what we’ll end up with.

$2x - 12$ becomes $2 (x - 6)$

$2x^2 - 4x - 48$ factored is $2 (x - 2x - 24$ and can still be reduced as $2 (x - 6) (x + 4)$ .

$x - 6$ stays as it is.

The second factored denominator is the least common denominator.

Instead of having the same denominator, we’ll just multiply each term by the least common denominator so we can cancel out later on.

$2 (x - 6) (x + 4) \times \dfrac{1}{2x - 12} + 2 (x - 6) (x+ 4) \times \dfrac{1}{2x^2 - 4x - 48} = 2 (x - 6) (x + 4) \times \dfrac{1}{x - 6}$

When multiplied, the equation remains the same since we didn’t do anything mathematically incorrect.

What we’ll do now is reduce.

At this point, we have no more denominators.

And we are left with

$x + 4 + 1 = 2 (x + 4)$

Now, we have a simpler equation we can solve.

Let’s combine like terms on the left side and distribute $2$ on the right side of the equation.

$x + 5 = 2x + 8$

Now, let’s get all the $x$ -es together.

$x - x + 5 = 2x - x + 8$

$5 = x + 8$

$5 - 8 = x + 8 - 8$

$-3 = x$

This is our final answer.

Another note for this is to always check our answer.

If we put the value of $x$ , we have to make sure that the value of the denominator does not equal to zero.

Because if the denominator is zero, that’s undefined.

Solving Rational Equations by using the Quadratic Formula

In the third video, we are going to solve rational equations by using the quadratic formula.

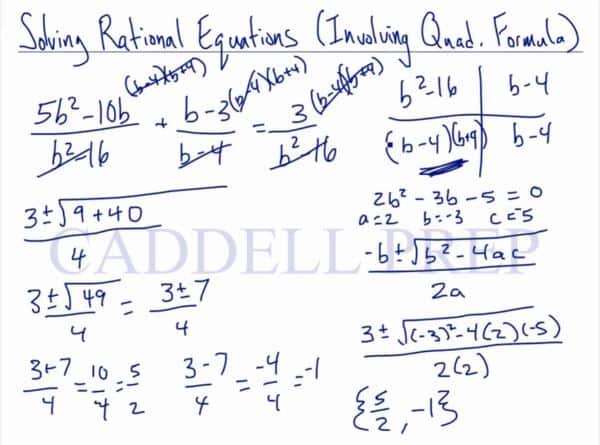
For example: $\frac{5b^2-10b}{b^2-16}+\frac{b-3}{b-4}=\frac{3}{b^2-16}$

Determine the LCD, which is $(b-4)(b+9)$ , and multiply it with each numerator
$\frac{(b-4)(b+9)5b^2-10b}{b^2-16}+\frac{(b-4)(b+9)b-3}{b-4}=\frac{(b-4)(b+9)3}{b^2-16}$

Reduce each expressions
$5b^2-10b+(b-3)(b-4)=3$

Use the distributive property
$5b^2-10b+b^2+4b-3b-12=3$

Combine like terms
$6b^2-9b-12=3$

Subtract 3 from both sides of the equation so that it is equal to 0
$6b^2-9b-15=0$

Divide by 3
$2b^2-3b-5=0$

Use the quadratic formula to find x. First, identify the values of a, b, and c
$a=2, b=-3, c=-5$

Substitute the values into the quadratic formula and solve
$\frac{-b\pm\sqrt{b^2-4ac}}{2a}$
$\frac{3\pm\sqrt{(-3)^2-4(2)(-5)}}{2(2)}$
$\frac{3\pm\sqrt{9-4(2)(-5)}}{2(2)}$
$\frac{3\pm\sqrt{9+40}}{4}$
$\frac{3\pm\sqrt{49}}{4}$
$\frac{3\pm7}{4}$

Solve $\frac{3\pm7}{4}$
$\frac{3+7}{4}$
$\frac{10}{4}$
$\frac{5}{2}$

$\frac{3-7}{4}$
$\frac{4}{4}$
$\frac{1}{1}$
$1$

x is $\frac{5}{2}$ and $-1$ , so the solution set is
$\{\frac{5}{2}, -1\}$

Examples of Solving Rational Equations by Getting LCD

Example 1

$\dfrac{x}{x^2+x-2}=\dfrac{x}{x^2+3x+2}-\dfrac{x}{x^2-1}$

First, let’s factor each of the denominator to find the LCD
$\dfrac{x}{(x-1)(x+2)}=\dfrac{x}{(x+1)(x+2)}-\dfrac{x}{(x+1)(x-1)}$
The LCD is $(x-1)(x+1)(x+2)$ , multiply the numerators by the LCD

$x(x+1)=x(x-1)-x(x+2)$

$x^2+x=x^2-x-x^2-2x$

$x^2+x=-3x$

Move the terms on each side to make one side equals to zero

$x^2+x+3x=0$

$x^2+4x=0$

Now, factor the expression
$x(x+4)=0$
We get:
$x=0$
and

$x+4=0$

$x=-4$

Therefore $x$ is $0$ and $-4$

Example 2

$\dfrac{2x-3}{x+1}-\dfrac{x}{x-1}=\dfrac{2x^2+7}{x^2-1}$
Determine the LCD, which is $(x+1)(x-1)$ , and multiply it with each numerator

$\dfrac{(x+1)(x-1)2x-3}{x+1}-\dfrac{(x+1)(x-1)x}{x-1}=\dfrac{(x+1)(x-1)2x^2+7}{x^2-1}$

$(x-1)(2x-3)-(x+1)(x)=2x^2+7$

$2x^2-5x+3-x^2+x=2x^2+7$

$x^2-4x+3=2x^2+7$

Subtract $x^2$ on both sides

$x^2-4x+3-x^2=2x^2+7-x^2$

$-4x+3=x^2+7$

Add $4x$ on both sides

$-4x+3+4x=x^2+7+4x$

$3=x^2+7+4x$

Subtract $3$ from both sides of the equation so that it is equal to $0$

$3-3=x^2+7+4x-3$

$0=x^2+4x+4$

Use the quadratic formula to find $x$ . First, identify the values of $a$ , $b$ , and $c$

$a=1,b=4, c=3$

$x=\dfrac{-b\pm\sqrt{b^2-4ac}}{2a}$

$x=\dfrac{-4\pm\sqrt{(4)^2-4(1)(3)}}{2(1)}$

$x=\dfrac{-4\pm\sqrt{16-12}}{2}$

$x=\dfrac{-4\pm\sqrt{4}}{2}$

$x=\dfrac{-4\pm2}{4}$

Solve $x=\dfrac{-4+2}{2}$

$x=\dfrac{-2}{2}$

$x=-1$

Solve $x=\dfrac{-4-2}{2}$

$x=\dfrac{-6}{2}$

$x=-3$

So the solution set is $\{-1, -3\}$

Video-Lesson Transcript

Let’s go over solving rational equations involving quadratic formula.

Let’s look at this equation

$\dfrac{5b^2 - 10b}{b^2 - 16} + \dfrac{b - 3}{b - 4} = \dfrac{3}{b^2 - 16}$

We have unlike denominators.

What we want is the common denominator. Or we’ll use a different method that can get rid of the denominators.

Let’s first examine the denominators.

$b^2 - 16$ is actually $(b - 4) (b + 4)$ .

$b - 4$ can’t be factored

So the least common denominator is $(b - 4) (b + 4)$

You can choose to multiply the second expression by $b + 4$ to have a common denominator.

Or multiply everything by the least common denominator then cancel the denominator out.

That’s what I’ll do.

Let me multiply everything by the least common denominator.

$(b - 4) (b + 4) \times \dfrac{5b^2 - 10b}{b^2 - 16} + (b - 4) (b + 4) \times \dfrac{b - 3}{b - 4} = (b - 4) (b + 4) \times \dfrac{3}{b^2 - 16}$

This is just my preferred method. But either methods will work.

Let’s cancel out now and we’re left with

$5b^2 - 10b + (b - 3) (b+4) = 3$

Make sure to put the parenthesis there.

You may be confused and only distribute $3$ when you have to distribute the whole thing.

Now, let’s first distribute the ones in the parenthesis.

$5b^2 - 10b + b^2 + 4b - 3b - 12 = 3$

Then let’s combine the like terms.

$6b^2 - 9b - 12 = 3$

Here, we have a quadratic.

So let’s get one side equal to zero.

$6b^2 - 9b - 12 - 3 = 3 - 3$

$6b^2 - 9b - 15 = 0$

Now, let’s factor this.

$3 (2b^2 - 3b - 5) = 0$

$3 \div 3 (2b^2 - 3b - 5) = \dfrac{0}{3}$

$2b^2 - 3b - 5 = 0$

We can do a number of ways to factor this.

But let me jump straight into using quadratic formula.

$\dfrac{-b \pm \sqrt{b^2 - 4ac}}{2a}$

Here $a = 2$ , $b = -3$ , and $c = -5$

Now, let’s substitute these values in.

$\dfrac{3 \pm \sqrt{(-3)^2 - 4 (2) (-5)}}{2 (2)}$

$\dfrac{3 \pm \sqrt{9 + 40}}{4}$

$\dfrac{3 \pm \sqrt{49}}{4}$

$\dfrac{3 \pm 7}{4}$

So we have two different answers.

$\dfrac{3 + 7}{4}$

$= \dfrac{10}{4}$

$= \dfrac{5}{2}$

and

$\dfrac{3 - 7}{4}$

$= \dfrac{-4}{4}$

$= -1$

So our solution set is

{ $\dfrac{5}{2}, -1$ }

Of course we have to check our answers.

Just to make sure that when we plug the values in, we won’t get zero in the denominator.

Just a quick look at the denominators, with our answers, plugged in.

Both of these should work out.